When we look at runners on the field, several aspects can be considered:
- Where they line up for a play
- Changing directions
- Running in an open field
Line-Up Positions
When we look at the positions of the backs, both offensive and defensive, we see that they typically line up away from the line of scrimmage on either side of the offensive and defensive linemen. Their positioning allows them room, or time, to accelerate from a state of rest and reach a high speed, to either run with the ball or pursue the ball carrier. Notice that the linebackers have far more room to accelerate than the linemen, and the wide receivers have far more room than the linebackers. So linebackers can reach higher speeds than linemen, and wide receivers can reach the highest speeds of all.
Changing Directions on the Field
Let's look at an example of a running play in which the quarterback hands the ball off to a running back. First, the running back starts from the set position, at rest, and accelerates to full speed (22 mi/h or 9.8 m/s) in 2 s after receiving the ball. His acceleration (a) is:
a = (vf - vo)/(tf - to)
a = (vf - vo)/(tf - to)
- vf is final velocity
- vo is initial velocity
- tf is final time
- to is initial time
a=(9.8 m/s - 0 m/s)/(2 s - 0 s)
a= 4.9 m/s2
As he runs with the flow of the play (e.g. to the right), he maintains constant speed (a = 0). When he sees an opening in the line, he plants his foot to stop his motion to the right, changes direction and accelerates upfield into the open. By planting his foot, he applies force to the turf. The force he applies to the turf helps to accomplish two things:
- Stop his motion to the right
- Accelerate him upfield
To stop his motion to the right, two forces work together. First, there is the force that he himself applies to the turf when he plants his foot. The second force is the friction between his foot and the turf. Friction is an extremely important factor in runners changing direction. If you have ever seen a football game played in the rain, you have seen what happens to runners when there is little friction to utilize. The following is what happens when a runner tries to change his direction of motion on a wet surface:
- As he plants his foot to slow his motion, the coefficient of friction between the turf and him is reduced by the water on the surface.
- The reduced coefficient of friction decreases the frictional force.
- The decreased frictional force makes it harder for him to stop motion his to the right.
- The runner loses his footing and falls.
The applied force and the frictional force together must stop the motion to the right. Let's assume that he stops in 0.5 s. His acceleration must be:
- a = (0 m/s - 9.8 m/s)/(0.5 s - 0 s)
- a = -19.6 m/s2
*The negative sign indicates that the runner is accelerating is in the opposite direction, to the left.
The force (F) required to stop him is the product of his mass (m), estimated at 98 kg (220 lbs), and his acceleration:
- F = ma = (98 kg)(-19.6 m/s2) = 1921 Newtons (N)
- 4.4 N = 1 lb
- F = ~500 lbs!
To accelerate upfield, he pushes against the turf and the turf applies an equal and opposite force on him, thereby propelling him upfield. This is an example of Isaac Newton's third law of motion, which states that "for every action there is an equal, but opposite reaction." Again, if he accelerates to full speed in 0.5 s, then the turf applies 1921 N, or about 500 lbs, of force. If no one opposes his motion upfield, he will reach and maintain maximum speed until he either scores or is tackled.
Running in an Open Field
When running in an open field, the player can reach his maximum momentum. Because momentum is the product of mass and velocity, it is possible for players of different masses to have the same momentum. For example, our running back would have the following momentum (p):
- p = mv = (98 kg)(9.8 m/s) = 960 kg-m/s
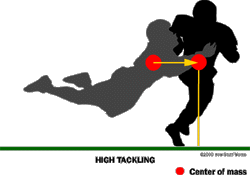
For a 125 kg (275 lb) lineman to have the same momentum, he would have to move with a speed of 7.7 m/s. Momentum is important for stopping (tackling, blocking) runners on the field.